Translate this page into:
Determinants of Under-Five Mortality in Rural Empowered Action Group States in India: An Application of Cox Frailty Model
✉Corresponding author email: rmpandey@yahoo.com.
-
Received: ,
Accepted: ,
Abstract
Objectives
In India there has been a decline in overall under-five mortality, with some states still showing very high mortality rates. It is argued that there is family clustering in mortality among children aged <5 years. We explored the effects of programmable (proximate) determinants on under-five mortality by accounting for family-level clustering and adjusting for background variables using Cox frailty model in rural Empowered Action Group states (EAG) in India and compared results with standard models.
Methods
Analysis included 13,785 live births that occurred five years preceding the National Family Health Survey-3 (2005-06). The Cox frailty model and the traditional Cox proportional hazards models were used.
Results
The Cox frailty model showed that mother's age at birth, place of delivery, sex of the baby, composite variable of birth order and birth interval, baby size at birth, and breastfeeding were significant determinants of under- five mortality, after adjusting for the familial frailty effect. The hazard ratio was 1.41 (95% CI=I.I4-I.75) for children born to mothers aged 12-19 years compared to mothers aged 20-30 years, 1.42 (95% CI=I.I2-I.79) for small-sized than average-sized babies at birth, and I02 (95% CI=8I-I28) for non-breastfed than breastfed babies. Children had significantly lower mortality risks in the richest than poorest wealth quintile. The familial frailty effect was 2.86 in the rural EAG states. The hazard ratios for the determinants in all the three models were similar except the death of a previous child variable in the Cox frailty model, which had the highest R2 and lowest log-likelihood.
Conclusions and Public Health Implications
While planning for the child survival program in rural EAG states, parental competence which explains the unobserved familial effect needs to be considered along with significant programmable determinants. The frailty models that provide statistically valid estimates of the covariate effects are recommended, when observations are correlated.
Keywords
Empowered Action Group States
Under-five mortality
National Family Health Survey
Frailty model
Unobserved familial effect
Programmable determinants
India
Introduction
Reducing under-five mortality is now a global concern. In 2001 as part of the Millennium Development goals (MDG) for health, nations pledged to ensure a two-thirds reduction in under-five mortality between 1990 and 2015[1] and at once a series of articles in Lancet by the Bellagio Study Group described various aspects of child survival[2,3,4,5,6]. Although under-five mortality is declining worldwide as a result of socioeconomic development and implementation of child survival interventions, nearly 8.8 million children die every year before their fifth birthday. India alone accounted for 21% of the world's under-five deaths occurring in 2008[7] owing to its large population. In India, states such as Assam, Arunachal Pradesh, Bihar, Chhattisgarh, Jharkhand, Madhya Pradesh, Orissa, Rajasthan, Uttar Pradesh, have higher Under-five mortality than the rest of India. The national average for under-five mortality is 74 per 1000[8]. The Ministry of Health and Family Welfare, India, established Empowered Action Group (EAG) in 2001 to have special focus by monitoring and facilitating the attainment of national health goals on some of these states which are demographically lagging behind. The EAG states constitute 45% of the total population of India and also have higher neonatal and infant mortality rates.
In developing countries, efforts have been made during the past three decades to reduce child mortality. Despite socioeconomic development and implementation of child survival interventions, prevailing high mortality may be due to the heterogeneity. This might have considerable implication for reproductive health and child survival programs[9]. Studies on determinants of child mortality have mainly used either logistic regression or Cox proportional hazards model assuming that the outcomes are independent. To find more accurate estimates for the determinants of child mortality that has critical implications for resource allocation for improving child survival, sibling structures in child mortality data from demographic surveys have been treated as multivariate failure time data[10,11,12,13]. As failure time data, many attempts have been made to extend the Cox proportional hazards model. In this context, the variance-corrected Cox model has received much attention[14,15]. In the variance-corrected Cox model, regression parameters of the determinants are estimated by ignoring intra-family correlation but adjusted for in the inference procedure; however, it ignores the variation of underlying risk among families. To overcome this, multivariate failure time data are modeled by an unobserved random quantity called frailties[16]. These frailties are common to observations from the same cluster and assumed to follow a given statistical distribution, known as multivariate random effects model or Cox frailty model.
In India, studies on child mortality have mainly addressed the role of maternal, socioeconomic and health-related determinants[9,17,18]. These studies were restricted to the analysis of mortality risks in children at individual level and not considered the correlation among children of the same family. We also want to emphasize those determinants which are nearer in time to the outcome and can be modified by program than those which are remote or far apart in time to the outcome of concern. The former covariates are referred to as programmable determinants and the latter as background variables. Therefore, we aimed to identify the programmable determinants of under-five mortality using Cox frailty model to account for sibling-level correlation for providing valid estimates needed for policy-decision making. In order to appreciate the influence of sibling-level correlation over the estimates of the determinants of under-five mortality, the results of Cox frailty model were compared with the Cox proportional hazards model and variance-corrected Cox model.
Methods
Data Sources
The third round of National Family Health Survey-3 (NFHS-3) in India was completed during 2005-06 covering a nationally representative sample of ever married women aged 15-49 years. This survey collected data on fertility, family planning, infant and child mortality, maternal and child health, etc. using a two-stage sample design in rural areas for each state of India. The first stage involved selection of primary sampling units, i.e., villages, with probability proportional to population size and the second stage involved systematic selection of households within each selected village[8]. The response rates for household and eligible women identified in the household were 98.5% and 95.5% respectively. The rural data of NFHS-3 for eight EAG states, viz., Bihar, Chhattisgarh, Jharkhand, Madhya Pradesh, Orissa, Rajasthan, Uttar Pradesh and Uttarakhand were combined and analyzed to identify the determinants of under-five mortality. In rural EAG states, retrospective maternity history was collected from 24,507 women aged 15-49 years. A total of 14,184 live births occurred within five years preceding the survey and mortality experience of 13,785 children were analyzed in this study. In 399 cases the information was missing on some of the variables used in the analysis. Of these 13,785 live births, 1,068 children died before reaching their fifth birthday.
Study Variables
The primary outcome, under-five mortality, was defined as time to death of a live born baby before his/her fifth birthday. Available potential predictors[19] of child survival as summarized in the conceptual framework of Mosley and Chen[20] was considered and grouped into programmable (proximate) determinants and background variables.
Programmable determinants included mother's age at birth, delivery assistance, place of delivery, mode of delivery, combined variable of birth order and birth interval, survival status of previous child, maternal subjective assessment of baby's size at birth, sex of the baby and ever breastfeeding; and the background variables included region (eight states), religion, caste, mother's education, mother's occupation, household wealth index, number of children in the family and desired time for pregnancy.
Analytical Models: Traditional Cox Proportional Hazards Model, Multivariate Cox Variance-Corrected and Frailty Models
The variance-corrected and frailty hazard models are multivariate not only in the usual sense of having multiple predictors, but also in the sense of having multiple responses, that is, responses from more than one child in the family.
Cox Proportional Hazards model:
Mathematically, it is written as
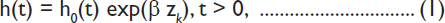
Where, h0(t) is an unspecified baseline hazard function and β denote the vector of the true regression coefficients for covariates zk, (k=1,2, &, p). We could obtain an estimator β of β based on the working assumption that the under-five deaths in each family were independent of one another.
Cox variance corrected model:
We supposed that conditional on covariate vector (zik), the marginal hazard function hik(t) for failure time of the kth child in the ith family, (k = 1,2,3,&.,Ki; i = 1,2,3,&,n) with the usual proportional hazards form and is given by
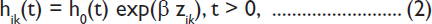
We could obtain an estimator β of β based on the working assumption that the under-five deaths in each family were independent of one another. But the equation (2) assumes that the births are related and hence adjusts for it in the inference, that is, the standard error by means of sandwich-type estimators[13] and so it is called as variance corrected models.
Cox frailty model
For the frailty model, we supposed that conditional on the frailty, v. the hazard function hik(t) for the failure time of the kth children in the ith family (k = 1,2,3,&.,K.; i = 1,2,3,&,n) follows the usual proportional hazards form and is given by:
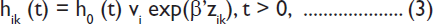
Where, vi, group-level (family) frailty. These frailties are unobservable, assumed to be independent and identically distributed with unit mean and unknown variance θ. Each family could have different values of random effects and the variability in the vis reflect heterogeneity of risks between families. If the variance of the random effect (frailty) is 0, then children from the same family are independent. The variance of the random effect lies between 0 and a. A larger variance implies greater heterogeneity in frailty across families and greater correlation among children belonging to the same family. The frailty (family) often assumed to follow gamma distribution for the sake of computational convenience and convergence[21,22,23] and this model is expected to yield correct z-ratios, on which researchers rely heavily for their conclusions[10].
Equations (2) and (3) reduce to the traditional Cox Proportional Hazards model[24], if the responses from each child in the family are assumed to be independent.
Statistical analysis
The complete data for all the EAG states were downloaded from Demographic Health Survey data distribution system website: http://www.measuredhs.com. All the variables were read and coded using Stata 9.0 (College Station, Texas, USA). The under-five mortality rate (U5MR) and its 95% CI with respect to potential determinants influencing under-five mortality was calculated. We identified potential determinants of under-five mortality using three models: the traditional Cox proportional hazards model, the variance-corrected Cox proportional hazards model, and the Cox frailty model. Univariate models were fitted followed by multivariate models. Programmable determinants were adjusted for background factors in the multivariate analysis in all the three models. The model performance was assessed using R-square and log-likelihood. The results were reported as hazard ratio (95% CI). The value of p<0.05 was considered statistically significant. The R-software (version 2.11.1,2010, The R foundation for Statistical Computing) was used to fit all the models.
Results
The trends in under-five mortality rates by major states in rural India for five years preceding the NFHS-1 (1992-92) and NFHS-3 (2005-06) are given in Table 1. Among the EAG states, no change in under-five mortality was found in Chhattisgarh and the highest decline in mortality (38.1%) was found in Bihar between the two surveys. The percentage decline in under-five mortality in rural India was 31.3 between the two surveys.
| Under-five mortality rates per 1000 live births for five years preceding the survey | |||
|---|---|---|---|
| States | NFHS-1 (1992-93) | NFHS-3 (2005-06) | Percentage Decline |
| Andhra Pradesh | 97.1 | 73.8 | 24.0 |
| Arunachal Pradesh* | 72.0 | 87.7 | -21.8 |
| Assam | 146.1 | 86.8 | 40.6 |
| Bihar | 139.2 | 86.2 | 38.1 |
| Chhattisgarh | 96.7 | 96.4 | 0.3 |
| Delhi* | 83.1 | 46.7 | 43.8 |
| Goa | 38.0 | 15.3 | 59.7 |
| Gujarat | 108.2 | 71.5 | 33.9 |
| Haryana | 107.0 | 61.2 | 42.8 |
| Himachal Pradesh | 71.0 | 44.7 | 37.0 |
| Jammu & Kashmir | 61.2 | 51.2 | 16.3 |
| Jharkhand | 112.8 | 101.2 | 10.3 |
| Karnataka | 94.4 | 61.5 | 34.9 |
| Kerala | 38.5 | 15.5 | 59.7 |
| Madhya Pradesh | 162.2 | 104.3 | 35.7 |
| Maharashtra | 81.1 | 58.7 | 27.6 |
| Meghalaya* | 86.9 | 70.5 | 18.9 |
| Mizoram* | 29.3 | 52.9 | -80.5 |
| Nagaland* | 20.7 | 64.7 | -212.6 |
| Manipur* | 61.7 | 41.9 | 32.1 |
| Orissa | 135.1 | 97.1 | 28.1 |
| Punjab | 71.8 | 53.0 | 26.2 |
| Rajasthan | 107.5 | 87.4 | 18.7 |
| Tamil Nadu | 98.0 | 43.2 | 55.9 |
| Tripura* | 104.6 | 59.2 | -43.4 |
| Uttarakhand | 96.4 | 65.1 | 32.5 |
| Uttar Pradesh | 154.2 | 100.0 | 35.1 |
| West Bengal | 104.0 | 64.1 | 38.4 |
| EAG States as a whole | 160.5 | 93.7 | 41.6 |
| India as a whole | 119.4 | 82.0 | 31.3 |
*Data represent under-five mortality rates for the complete states
The distribution of live births by family is shown in Table 2. More than one third (41%) of the families contributed two or more children to the sample. About 40 percent of the total 13,785 children did not have sibling. A total of 1,068 under-five deaths occurred to 969 (10%) families.
| Children | Deaths per Family | Total | Percent of Total Children | Percent of Total Deaths | |||
|---|---|---|---|---|---|---|---|
| 0 | 1 | 2 | 3 | ||||
| 1 | 5,297 | 263 | 5,560 | 40.3 | 24.6 | ||
| 2 | 2,768 | 438 | 37 | 3,243 | 47.1 | 47.9 | |
| 3 | 322 | 163 | 33 | 0 | 518 | 1 1.3 | 21.4 |
| 4 | 11 | 11 | 17 | 6 | 45 | 1.3 | 5.9 |
| 5 | 0 | 1 | 0 | 0 | 1 | 0.0 | 0.7 |
| Total | 8,398 | 876 | 87 | 6 | 9,367 | 100.0 | 100.0 |
| Percent of total children | 85.9 | 12.2 | 1.7 | 0.2 | 100.0 | ||
| Percent of total deaths | 0.0 | 82.0 | 16.3 | 1.7 | 100.0 | ||
The number of live births and under-five mortality with respect to the background factors and programmable determinants are shown in Table 3. One third of the total live births were from Uttar Pradesh; only 36.4 percent live births belong to scheduled caste (21.3%) and scheduled tribes (15.1 %) and mothers of two-thirds of the live births were illiterate. The under-five mortality (per 1,000 live births) was 86.9 in Uttar Pradesh, 89.9 among scheduled caste mothers, 86.7 among illiterate mothers, 81.7 among families with more than two children, 87.9 in the poorest wealth quintile, 104.2 among children born to mothers' aged 12-19 years, 139.3 in mothers having previous birth interval of less than two years and parity more than three, 107 among small sized babies, and 143 among children with history of dead sibling, which were having very high under-five mortality than their counterparts.
| Background Variables | Live Birth (n = 13,785) | Under-Five mortality (95% CI) | Programmable Determinants | Live Births (n = 13,785) | Under-Five mortality (95% CI) |
|---|---|---|---|---|---|
| EAG States | 13,785 (100.0) | Mother's age at birth | 24.1±5.5 | ||
| Bihar | 1,562 (11.3) | 65.9 (53.6,78.3) | 12-19 y | 2,868 (20.8) | 104.2 (93.1,115.4) |
| Jharkhand | 1,148 (8.3) | 73.2 (58.1,88.3) | 20-30 y | 9,055 (65.7) | 69.1 (63.9,74.4) |
| Madhya Pradesh | 1,738 (12.6) | 86.3 (73.1,99.5) | ≥31 y | 1,862 (13.5) | 76.8 (64.7,88.9) |
| Chhattisgarh | 1,162 (8.4) | 78.3 (62.8,93.8) | Place of Delivery | ||
| Rajasthan | 1,500 (10.9) | 74.0 (60.7,87.3) | Institutional | 2,391 (17.3) | 75.3 (64.7,85.9) |
| Orissa | 1,220 (8.9) | 72.1 (57.6,86.7) | Non-institutional | 11,394 (82.7) | 77.9 (73.0,82.9) |
| Uttar Pradesh | 4,587 (33.3) | 86.9 (78.8,95.1) | Delivery assistance | ||
| Uttarakhand | 868 (6.3) | 48.4 (34.0,62.7) | Doctors/ANM/HP | 3,582 (26.0) | 68.4 (60.1,76.7) |
| Caste | DAI/TBA | 6,783 (49.2) | 79.0 (72.6,85.4) | ||
| Scheduled Caste | 2,938 (21.3) | 89.9 (79.5,100.2) | Relatives/no one | 3,420 (24.8) | 83.9 (74.6,93.2) |
| Scheduled Tribe | 2,085 (15.1) | 88.2 (76.1,100.4) | Type of Delivery | ||
| Others | 8,762 (63.6) | 70.8 (65.4,76.1) | Normal | 13,439 (97.5) | 77.7 (73.2,82.2) |
| Religion | Caesarian | 346 (2.5) | 69.4 (42.5,96.3) | ||
| Hindu | 11,913(86.4) | 76.9 (72.2,81.8) | Sex of the baby | ||
| Others | 1,872 (13.6) | 80.7 (68.3,93.0) | Male | 7,073 (51.3) | 71.3 (65.3,77.3) |
| Mother's education | Female | 6,712(48.7) | 84.0 (77.4,90.7) | ||
| Illiterate | 9,226 (66.9) | 86.7 (80.9,92.5) | Birth Order & Birth Interval | ||
| Primary and above | 4,559 (33.1) | 58.8 (51.9,65.6) | First Birth Order | 3,340 (24.2) | 94.0 (84.1,103.9) |
| Mother's occupation | 2-3 Birth Order & Birth Interval ≥ 2y | 3,651 (26.5) | 46.3 (39.5,53.1) | ||
| Profess ional/clerical/sales | 213 (1.6) | 70.4 (35.8,105.1) | 2-3 Birth Order & Birth Interval < 2y | 1,838 (13.3) | 91.4 (78.2,104.6) |
| Agri re 1 ated/u nski 1 led | 6,508 (47.2) | 79.3 (72.7,85.9) | ≥ 4 Birth Order & Birth Interval ≥ 2y | 3,405 (24.7) | 59.0 (51.1,66.9) |
| Not working | 7,064 (51.2) | 76.0 (69.8,82.2) | ≥ 4 Birth Order & Birth Interval < 2 y | 1,551 (11.3) | 139.3 (122.0,156.5) |
| Total number of children | Size of the baby at birth | ||||
| ≤2 | 5,139 (37.6) | 70.4 (63.4,77.4) | Very small & small | 3,064 (22.2) | 107.0 (96.1,118.0) |
| >2 | 8,646 (62.7) | 81.7 (75.9,87.4) | Average | 8,182 (59.3) | 68.4 (62.9,73.9) |
| Household Wealth Index | Very large & large | 2,539 (18.4) | 70.9 (60.9,80.9) | ||
| Poorest | 5,637 (40.9) | 87.9 (80.6,95.4) | Survival status of previous child | ||
| Poorer | 3,714(26.9) | 85.1 (76.1,94.1) | Alive | 9,264 (67.2) | 63.1 (58.2,68.1) |
| Middle | 2,440 (17.7) | 70.9 (60.7,81.1) | First Baby | 3,340 (24.2) | 94.0 (84.1,103.9) |
| Richer | 1,485 (10.8) | 43.1 (32.8,53.4) | Dead | 1,181 (8.6) | 143.1 (123.1,163.1) |
| Richest | 509 (3.7) | 37.3 (20.8,53.9) | Breastfeeding | ||
| Desire for pregnancy | Yes | 13,152 (95.4) | 54.3 (50.4,58.2) | ||
| Then | 10,699 (77.6) | 76.2(71.2,81.3) | No | 633 (4.6) | 559 (520,598) |
| Later | 1,284 (9.3) | 72.4 (58.2,86.6) | |||
| No more | 1,802 (13.1) | 88.2 (75.1,101.3) | |||
The results of programmable determinants of under-five deaths adjusting for the background variables using all the three models are given in Table 4. The estimates are exactly the same in Models 1 and 2; only standard errors are corrected in Model 2, and in Model 3, both estimates and standard errors are corrected. The determinants found to be significant in Model 1 were also significant in Model 3 except death of a previous child and in Model 2 except mother's age at birth. In the frailty model, the mortality hazards for children born to mothers aged 12-19 years at birth were 1.41 (95% CI: 1.14,1.75) times higher than children born to mothers aged 20-30 years at birth and in the variance-corrected model, the hazard ratio (1.19) for the same variable was not statistically significant. The mortality hazard for the female child has increased from 17% to 22% when unobserved familial effect is taken into account. Small size babies at birth had 42% excess hazard than the average size babies at birth. The mortality hazards for first-born children and fourth-or-higher birth order children with preceding birth interval of less than two years were 2.04 (95% CI: 1.52,2.73) and 2.42 (95% CI: 1.84,3.18) times the hazard for second or third birth order children with a longer birth interval (p< 0.001). Infants who were not breastfed had significantly higher hazard of death (HR = 102; 95% CI: 81,128) than those who were breastfed. The hazard ratio was 44% lower in non-institutional than institutional deliveries.
| Programmable | Model 1 (Cox Proportional Hazards Model) | Model 2 (CoxVariance Corrected Model) | Model 3 (Cox Frailty Model) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Determinants | Unadjusted | Adjusteda | Pb | Unadjusted | Adjusteda | Pb | Unadjusted | Adjusteda | Pb |
| Mother's age at birth (y) | |||||||||
| 12-19 y | 1.53 (1.33,1.76) | 1.19(1.00,1.41) | 0.048 | 1.53 (1.33,1.76) | 1.19 (0.98,1.44) | 0.078 | 1.57 (1.36,1.82) | 1.41 (1.14,1.75) | 0.002 |
| ≥ 31 y | 1.11 (0.92,1.33) | 1.04 (0.85,1.27) | 0.715 | 1.11 (0.91,1.34) | 1.04 (0.84,1.29) | 0.733 | 1.09 (0.90,1.32) | 0.96 (0.75,1.24) | 0.770 |
| Place of Delivery | |||||||||
| Non-institutional | 1.01 (0.86,1.18) | 0.69 (0.52,0.93) | 0.014 | 1.01 (0.85,1.19) | 0.69 (0.51,0.95) | 0.021 | 1.00 (0.84,1.18) | 0.66 (0.46,0.94) | 0.021 |
| Delivery assistance by whom | |||||||||
| DAI/TBA | 1.13 (0.97,1.31) | 1.13 (0.87,1.46) | 0.353 | 1.13 (0.96,1.32) | 1.13 (0.86,1.48) | 0.375 | 1.13 (0.97,1.33) | 1.33 (0.97,1.81) | 0.075 |
| Relatives/no one | 1.21 (1.02,1.43) | 1.25 (0.95,1.65) | 0.115 | 1.21 (1.01,1.44) | 1.25 (0.94,1.67) | 0.130 | 1.20 (1.01,1.44) | 1.38 (0.99,1.93) | 0.061 |
| Type of Delivery | |||||||||
| Caesarian | 0.92 (0.62,1.38) | 1.01 (0.66,1.56) | 0.948 | 0.92 (0.60,1.41) | 1.01 (0.65,1.58) | 0.949 | 0.94 (0.62,1.44) | 0.89 (0.51,1.55) | 0.680 |
| Sex of the baby | |||||||||
| Female | 1.18 (1.05,1.34) | 1.17 (1.03,1.32) | 0.013 | 1.18 (1.05,1.34) | 1.17 (1.02,1.33) | 0.021 | 1.19 (1.05,1.34) | 1.22 (1.05,1.41) | 0.008 |
| Birth Order (BO) & Birth Interval (Bl) | |||||||||
| First BO | 2.10 (1.74,2.53) | 2.03 (1.58,2.61) | <0.001 | 2.10 (1.74,2.53) | 2.03 (1.54,2.68) | <0.001 | 2.14 (1.77,2.59) | 2.04 (1.52,2.73) | <0.001 |
| 2-3 BO & Bl < 2y | 1.99 (1.61,2.46) | 1.64 (1.31,2.04) | <0.001 | 1.99 (1.60,2.47) | 1.64 (1.28,2.09) | <0.001 | 1.94 (1.56,2.41) | 1.97 (1.52,2.57) | <0.001 |
| ≥ 4 BO & Bl ≥ 2y | 1.27 (1.04,1.56) | 1.04 (0.82,1.30) | 0.766 | 1.27 (1.04,1.57) | 1.04 (0.82,1.31) | 0.773 | 1.26 (1.02,1.55) | 1.06 (0.80,1.39) | 0.700 |
| ≥ 4 BO & Bl < 2 y | 3.04 (2.49,3.72) | 2.31 (1.85,2.89) | <0.001 | 3.04 (2.47,3.74) | 2.31 (1.82,2.93) | <0.001 | 2.93 (2.37,3.61) | 2.42 (1.84,3.18) | <0.001 |
| Size of the baby at birth | |||||||||
| Very small & small | 1.58 (1.31,1.89) | 1.39 (1.16,1.68) | <0.001 | 1.58 (1.31,1.90) | 1.39 (1.14,1.70) | 0.001 | 1.62 (1.34,1.96) | 1.42 (1.12,1.79) | 0.003 |
| Average | 0.96 (0.82,1.14) | 0.99 (0.83,1.17) | 0.871 | 0.96(0.81,1.14) | 0.99 (0.82,1.19) | 0.880 | 0.96 (0.80,1.14) | 0.97 (0.78,1.19) | 0.740 |
| Survival status of previous child | |||||||||
| Dead | 2.40 (2.02,2.84) | 1.86 (1.56,2.21) | <0.001 | 2.40 (2.03,2.83) | 1.86 (1.54,2.24) | <0.001 | 2.22 (1.86,2.64) | 0.82 (0.67,1.02) | 0.072 |
| Breastfeeding | |||||||||
| No | 16.6(14.6,18.8) | 18.2(15.9,20.9) | <0.001 | 16.6(14.3,19.2) | 18.2(15.5,21.4) | <0.001 | 116.0 (93,146) | 102.0 (81,128) | <0.001 |
| Background Variables EAG States | |||||||||
| Bihar | 1.37 (0.95,1.95) | 1.55 (1.06,2.26) | 0.022 | 1.37 (0.95,1.96) | 1.55 (1.00,2.41) | 0.049 | 1.39 (0.95,2.02) | 1.63 (0.98,2.74) | 0.063 |
| Jharkhand | 1.52 (1.50,2.20) | 1.77 (1.19,2.63) | 0.005 | 1.52 (1.04,2.23) | 1.77(1.11,2.81) | 0.016 | 1.52 (1.03,2.24) | 1.49 (0.87,2.56) | 0.140 |
| Madhya Pradesh | 1.79 (1.27,2.52) | 1.98 (1.38,2.85) | <0.001 | 1.79 (1.27,2.54) | 1.98 (1.29,3.04) | 0.002 | 1.80 (1.26,2.58) | 2.29 (1.39,3.78) | 0.001 |
| Chhattisgarh | 1.63 (1.13,2.35) | 2.53 (1.71,3.75) | <0.001 | 1.63 (1.13,2.36) | 2.53 (1.62,3.96) | <0.001 | 1.65 (1.13,2.43) | 2.47(1.46,4.20) | <0.001 |
| Rajasthan | 1.54 (1.08,2.20) | 2.12 (1.47,3.08) | <0.001 | 1.54 (1.08,2.20) | 2.12 (1.39,3.24) | <0.001 | 1.55 (1.07,2.24) | 2.06 (1.24,3.41) | 0.005 |
| Orissa | 1.50 (1.04,2.17) | 1.56 (1.05,2.28) | 0.027 | 1.50 (1.06,2.18) | 1.55 (0.99,2.42) | 0.056 | 1.50 (1.02,2.20) | 1.88 (1.10,3.22) | 0.021 |
| Uttar Pradesh | 1.81 (1.32,2.50) | 2.51 (1.80,3.51) | <0.001 | 1.82 (1.32,2.50) | 2.51 (1.69,3.72) | <0.001 | 1.82 (1.31,2.54) | 2.41 (1.51,3.85) | <0.001 |
| Caste | |||||||||
| Scheduled Caste | 1.28 (1.11,1.48) | 1.09 (0.94,1.27) | 0.251 | 1.28 (1.10,1.49) | 1.09 (0.93,1.29) | 0.293 | 1.28 (1.10,1.49) | 1.18 (0.96,1.44) | 0.110 |
| Scheduled Tribe | 1.25 (1.06,1.47) | 1.26 (1.04,1.52) | 0.017 | 1.25 (1.05,1.48) | 1.26 (1.02,1.55) | 0.029 | 1.25 (1.05,1.49) | 1.38 (1.08,1.76) | 0.011 |
| Religion | |||||||||
| Others | 1.04 (0.88,1.24) | 1.09 (0.90,1.32) | 0.364 | 1.04 (0.87,1.24) | 1.09 (0.88,1.35) | 0.420 | 1.05 (0.87,1.26) | 1.24 (0.97,1.59) | 0.080 |
| Mother's education | |||||||||
| Illiterate | 1.47 (1.28,1.69) | 1.27 (1.09,1.49) | 0.003 | 1.47 (1.27,1.70) | 1.27 (1.06,1.53) | 0.010 | 1.48 (1.28,1.71) | 1.29 (1.05,1.59) | 0.014 |
| Mother's occupation | |||||||||
| Agri related/unskilled | 1.14(0.68,1.91) | 0.90 (0.53,1.51) | 0.683 | 1.14 (0.64,2.05) | 0.90 (0.47,1.72) | 0.744 | 1.17(0.68,2.01) | 0.80 (0.41,1.50) | 0.500 |
| Not working | 1.11 (0.67,1.86) | 0.89 (0.53,1.50) | 0.670 | 1.11 (0.62,1.99) | 0.89 (0.47,1.71) | 0.733 | 1.13 (0.65,1.94) | 0.80 (0.41,1.54) | 0.500 |
| Total number of children | |||||||||
| >2 | 1.11 (0.97,1.25) | 1.27 (1.00,1.53) | 0.047 | 1.11 (0.97,1.26) | 1.24 (0.97,1.59) | 0.086 | 1.08 (0.94,1.23) | 1.40 (1.08,1.81) | 0.009 |
| Household Wealth Index | |||||||||
| Poorer | 0.97 (0.84,1.11) | 0.97 (0.84, 1.13) | 0.729 | 0.97 (0.83,1.12) | 0.97 (0.83,1.15) | 0.753 | 0.97 (0.83,1.12) | 1.03 (0.85,1.25) | 0.760 |
| Middle | 0.80 (0.68,0.95) | 0.84 (0.69,1.02) | 0.077 | 0.80 (0.67,0.96) | 1.84 (0.68,1.04) | 0.104 | 0.80 (0.66,0.96) | 0.81 (0.64,1.05) | 0.110 |
| Richer | 0.48 (0.37,0.63) | 0.54 (0.40,0.72) | <0.001 | 0.48 (0.37,0.64) | 0.54 (0.39,0.74) | <0.001 | 0.48 (0.37,0.63) | 0.45 (0.31,0.66) | <0.001 |
| Richest | 0.42 (0.27,0.67) | 0.53 (0.32,0.86) | 0.011 | 0.42 (0.26,0.69) | 0.53 (0.31,0.90) | 0.018 | 0.42 (0.26,0.71) | 0.40 (0.21,0.75) | 0.005 |
| Desire for pregnancy | |||||||||
| Later | 0.96 (0.77,1.19) | 0.88 (0.70,1.10) | 0.25 | 0.96 (0.76,1.21) | 0.88 (0.67,1.15) | 0.348 | 0.98 (0.78,1.23) | 1.17 (0.89,1.53) | 0.270 |
| No more | 1.17 (0.98,1.38) | 1.17 (0.96,1.42) | 0.113 | 1.17(0.97,1.41) | 1.17 (0.95,1.45) | 0.147 | 1.19 (1.00,1.43) | 1.22 (0.96,1.56) | 0.100 |
| Variance of frailty | 2.86 | <0.001 | |||||||
| Log likelihood/l-likelihood | -9246.9 | -9246.9 | -9150.5 | ||||||
| R2 | 10.80% | 10.80% | 27.4% | ||||||
Reference categories: Mother's age at birth 20-30 y, institutional Delivery, Delivery assistance by Doctors/ANM/HP, Male children, 2-3 Birth Order & Birth Interval ≥ 2y, Very large & large size of the baby at birth, surviving previous sibling, breastfed children, Uttarakhand state, other caste, Hindu religion, literate mothers, Professional/Clerical/Sales,≤ 2 children, poorest household wealth index and then for desire for pregnancy;Adjusted for background factors such as EAG States, religion, caste, mother's education, mother's occupation, number of children, wealth Index, desire time for pregnancy and other determinants; pb- p-value for multivariate analysis and p<0.05, statistically significant.
EAG states as a background variable was significantly associated with under-five mortality. The State, Uttarakhand, was selected as the reference category due to low under-five mortality rates among the EAG states. The hazard ratios were increased in all the EAG states except Jharkhand after adjustment for programmable determinants and other background variables. However, the adjusted hazard ratios were statistically significant for only Madhya Pradesh, Chhattisgarh, Rajasthan, Orissa and Uttar Pradesh. The other background variables such as caste, mother's education and household wealth index were significantly associated with under-five mortality as shown in Table 4.
Most hazard ratios for the proximate determinants are similar across the three types of models but the most notable finding is the change in the effect of the death of a previous child variable. The multiplicative effect of this variable changes from an 86% excess risk to an 18% reduction in risk (albeit not statistically significant) when unobserved familial effect is taken into account as a gamma frailty. The gamma frailty is 2.86 which means that larger unmeasured familial effect is present and is statistically significant (p<0.001).
In general, the z-statistics (not shown here) are found to be smaller in the random-effects/frailty model than in the traditional Cox and variance-corrected Cox models except for some of the covariates.
The R2 and log likelihood/I-likelihood are preferred for comparing the three models. The Cox frailty model was considered the best model as it had the highest R2 and lowest log likelihood compared to the other two models.
Discussion
The primary goal of the study was to assess the determinants of under-five mortality by applying an appropriate model to account for sibling-level correlation and thus provide valid estimates for correct statistical inference needed for policy-decision making. We found that children born in Chhattisgarh had higher risk of dying before age five, followed by children born in Uttar Pradesh and Madhya Pradesh. These states require health interventions that target under-five mortality reduction, particularly in rural areas. Next, mother's education and wealth index emerge as powerful background covariates of under-five mortality in the EAG states, for the reason that both are known to be associated with better child care practices. Thus, the study urges the policy makers to focus on educating illiterate mothers about the child care; however, policy aiming at improving maternal education and poverty reduction is needed for sustainability.
We know that changes in the z statistics depend on the size of the parameter estimates along with the magnitude of the standard error. In general the z-statistics are smaller in magnitude in frailty model as compared to other models which we also observed in our results, clearly indicating that the sample of correlated observations contains less information than the independent sample. We also observed higher z-statistics for some covariates as observed by Sastry[12], for example, mother's age at birth of 12-19 y (ZModel 3 = 3.13 vs. Z Model 1= 1.98) in the Cox frailty model than the traditional Cox model.
The assumption of the Cox Proportional Hazards model is likely to be incorrect if we suspect that siblings share environmental or genetic influences beyond explicit covariates included in the model[11]. To account for this correlation, if we correct the standard error alone, it might lead to the biased inference, casting doubt especially on the more marginally significant results. The covariate, maternal age 12-19 years at child birth, was found to be marginally significant in the traditional Cox model and was statistically not significant in the variance-corrected Cox model. However, this variable was highly significant in the Cox frailty model which reiterates the importance of simultaneous correction of both parameter estimates and the standard error when analyzing correlated observations.
The next interesting aspect of the paper is estimates of the observed covariate effects. There were remarkably stable in all the three models except survival status of previous child variable. This has been already noted in previous studies[10,11,12] that the positive effect of this variable indeed acts as a proxy in the traditional Cox model. As pointed out by Guo and Rodríguez G[11], the hazard ratio of less than one in frailty model suggests that the death of a previous child lowers the risk of the surviving siblings through less competition for family resources or inducing changes in the parental behavior since death is a traumatic event. A non-protective role of institutional deliveries in the present study was found as pointed out by Titaley et al[25] and this might be complicated deliveries brought to the institution with three delays[26].
Estimation of family influences is difficult in that familial effects other than general socioeconomic status are very difficult to observe. Clustering of deaths in families was explained in rural Punjab[9] and in Guatemalan families[10] by household's economic status and mother's education. We found high variance of unobserved familial effect of 2.86 in the rural area of EAG states even after taking into account all possible cultural and socio-economic variables. This large unobserved heterogeneity at family level could be a result of greater variability in child care practices, health care and mother's personal abilities[18]. Also the female child is more likely to die before reaching age five than the male child which might be related to behavioral and environmental factors[5,27]. Thus, parental competence, genetic and other factors like nutritional deficiency, personal illness of the child etc which were not included in the present study might be the explanation for the family frailty in these rural EAG states.
The strengths of this study are the use of nationally representative survey of NFHS-3 (2005-06) data and the application of the Cox frailty model to estimate unbiased parameter estimates for determinants after accounting for familial effect. However, the cross-sectional nature of our study is its main limitation. The study should therefore be interpreted with caution. The variable, breastfeeding, was not considered as a time-dependent covariate due to methodological difficulty of the frailty model.
Conclusions and Public Health Implications
In conclusion, this paper confirms the hypothesis that the risk of under-five death among families is heterogeneous and identifies determinants associated with under-five deaths. Many determinants can be modified by child survival programs to enhance child survival, such as intensive antenatal and delivery care to young pregnant women and women having parity of more than two with preceding birth interval of less than two years; providing ideal nutritional supplement to infants who are small and or very small at the time of birth; improving mother's child care practices by health education if mother has lost previous child; and reemphasizing exclusive breastfeeding for six months with introduction of complementary feeding at appropriate time. In the setting of correlated observations, the Cox frailty models are recommended for providing statistically valid estimates of the effects of proximate determinants after adjusting for the background variables and unobserved random effects.
Acknowledgements:
We acknowledge the National Family Health Survey India, International Institute of Population Sciences, Mumbai, for data collection and providing us data for analysis. We also acknowledge Dr. Padam Singh, Dr. P. P. Talwar, Dr. Bir Singh and Dr. Lalitendu Jagatdeb for their guidance and advice in the work.
Conflicts of Interest:
None.
References
- Road Map towards the implementation of the United Nations millennium declaration: report of the Secretary-General (UN document no. A/56/326) New York: United Nations; 2001.
- [Google Scholar]
- Where and why are 10 million children dying every year? Lancet. 2003;361(9376):2226-2237.
- [CrossRef] [PubMed] [Google Scholar]
- How many child deaths can we prevent this year? Lancet. 2003;362(9377):65-71.
- [CrossRef] [PubMed] [Google Scholar]
- The Multi-Country Evaluation of IMCI Study Group. Reducing child mortality: can public health deliver? Lancet. 2003;362(9378):159-164.
- [CrossRef] [PubMed] [Google Scholar]
- Applying an equity lens to child health and mortality: more of the same is not enough. Lancet. 2003;362(9379):233-241.
- [CrossRef] [PubMed] [Google Scholar]
- Knowledge into action for child Survival. Lancet. 2003;362(9380):323-327.
- [CrossRef] [PubMed] [Google Scholar]
- Levels and trends in under-5 mortality, 1990-2008. Lancet. 2010;375(9709):100-103.
- [CrossRef] [PubMed] [Google Scholar]
- 2007 National Family Health Survey (NFHS-3), 2005-06. India: Volume 1. Mumbai: IIPS;
- [Google Scholar]
- Socio-economic status and clustering of child deaths in rural Punjab. Vol 51. Population Studies; 1997. p. :191-202.
- [CrossRef] [Google Scholar]
- Use of sibling data to estimate family mortality effects in Guatemala. Demography. 1993;30(1):15-32.
- [CrossRef] [PubMed] [Google Scholar]
- Estimating a multivariate proportional hazards model for clustered data using the EM algorithm with an application to child survival in Guatemala. Journal of the American Statistical Association. 1992;87(420):969-976.
- [CrossRef] [Google Scholar]
- A nested frailty model for survival data, with an application to the study of child survival in northeast Brazil. Journal of the American Statistical Association. 1997;92(438):426-435.
- [CrossRef] [PubMed] [Google Scholar]
- Survival analysis for unobserved heterogeneity on estimated mortality in Taiwan. Economics Bulletin. 2008;9(25):1-10.
- [Google Scholar]
- Marginal regression models for multivariate failure time data. Journal of the American Statistical Association. 1998;93(443):1164-1175.
- [CrossRef] [Google Scholar]
- Cox-type regression analysis for large numbers of small groups or correlated failure time observations, survival analysis: state of the art, J. P. Klein and P. K. Goel. Kluwer Academic Publishers; 1992. p. :237-247.
- [CrossRef] [Google Scholar]
- The impact of heterogeneity in individual frailty on the dynamics of mortality. Demography. 1979;16(3):439-454.
- [CrossRef] [PubMed] [Google Scholar]
- Components of under-five mortality trends, current stagnation and future forecasting levels. National Commission on Macroeconomics and Health Background papers-Burden of Disease in India. Ministry of Health and Family Welfare, Government of India. 2005
- [Google Scholar]
- Death clustering, mothers' education and the determinants of child mortality in rural Punjab, India. Population Studies. 1990;44(3):489-505.
- [CrossRef] [Google Scholar]
- Factors associated with trends in infant and child mortality in developing countries during the 1990s. Bulletin of World health Organization. 2000;78(10):1256-1270.
- [Google Scholar]
- An analytical framework for the study of child survival in developing countries. Population and Development Review. 1984;10(Suppl):24-45.
- [CrossRef] [Google Scholar]
- Semiparametric estimation of random effects using the Cox model based on the EM algorithm. Biometrics. 1992;48(3):795-806.
- [CrossRef] [PubMed] [Google Scholar]
- Asymptotic theory for the correlated gamma-frailty model. Annals of Statistics. 1998;26(1):183-214.
- [CrossRef] [Google Scholar]
- Modeling survival data. In: Extending the Cox model. Berlin: Springer-Verlag; 2000.
- [CrossRef] [Google Scholar]
- Regression models and life tables. Journal of the Royal Statistical Society, Series B. 1972;74(2):187-220.
- [CrossRef] [Google Scholar]
- Type of delivery attendant, place of delivery and risk of early neonatal mortality: analyses of the 1994-2007 Indonesia Demographic and Health Surveys. Health Policy and Planning 2011 [Epub ahead of print]
- [CrossRef] [PubMed] [Google Scholar]
- Too far to walk: maternal mortality in context. Social Science in Medicine. 1994;38(8):1089-1110.
- [CrossRef] [PubMed] [Google Scholar]
- Reducing child mortality in India in the new millennium. Bulletin of the World Health Organization. 2000;78(10):1192-1199.
- [Google Scholar]





